Knaster–Kuratowski–Mazurkiewicz lemma
Knaster–Kuratowski–Mazurkiewicz lemma

The Knaster–Kuratowski–Mazurkiewicz lemma is a basic result in mathematical fixed-point theory published in 1929 by Knaster, Kuratowski and Mazurkiewicz.[1]
The KKM lemma can be proved from Sperner's lemma and can be used to prove the Brouwer fixed-point theorem.
Statement
 be a
be a -dimensionalsimplexwith nverticeslabeled as
-dimensionalsimplexwith nverticeslabeled as .
. ofclosed setssuch that for any
ofclosed setssuch that for any , theconvex hullof the vertices corresponding to
, theconvex hullof the vertices corresponding to iscoveredby
iscoveredby .
.The KKM lemma says that a KKM covering has a non-empty intersection, i.e:
 .
.Example
 may serve as an illustration. In this case the simplex
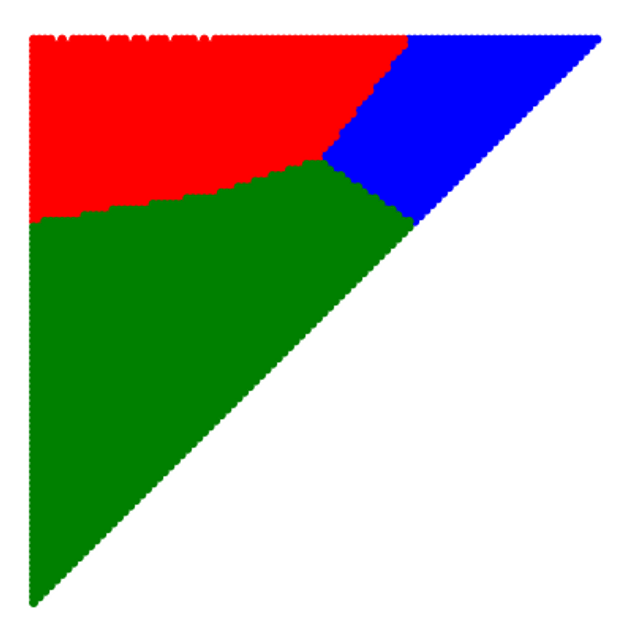
may serve as an illustration. In this case the simplex is a triangle, whose vertices we can label 1, 2 and 3. We are given three closed sets
is a triangle, whose vertices we can label 1, 2 and 3. We are given three closed sets such that:
such that:covers vertex 1, covers vertex 2, covers vertex 3.
The edge 12 (from vertex 1 to vertex 2) is covered by the sets and , the edge 23 is covered by the sets and , the edge 31 is covered by the sets and .
The union of all three sets covers the entire triangle
 have at least one point in common.
have at least one point in common.The lemma is illustrated by the picture on the right, in which set #1 is blue, set #2 is red and set #3 is green. The KKM requirements are satisfied, since:
Each vertex is covered by a unique color.
Each edge is covered by the two colors of its two vertices.
The triangle is covered by all three colors.
The KKM lemma states that there is a point covered by all three colors simultaneously; such a point is clearly visible in the picture.
Equivalent results
There are several fixed-point theorems which come in three equivalent variants: an algebraic topology variant, a combinatorial variant and a set-covering variant. Each variant can be proved separately using totally different arguments, but each variant can also be reduced to the other variants in its row. Additionally, each result in the top row can be deduced from the one below it in the same column.[2]
| Algebraic topology | Combinatorics | Set covering |
|---|---|---|
| Brouwer fixed-point theorem | Sperner's lemma | Knaster–Kuratowski–Mazurkiewicz lemma |
| Borsuk–Ulam theorem | Tucker's lemma | Lusternik–Schnirelmann theorem |
Generalizations
Permutations
 . Then, there exists a permutation
. Then, there exists a permutation of the coverings with a non-empty intersection, i.e:
of the coverings with a non-empty intersection, i.e: .
.Gale wrote about his lemma: "A colloquial statement of this result is the red, white and blue lemma which asserts that if each of three people paint a triangle red, white and blue according to the KKM rules, then there will be a point which is in the red set of one person, the white set of another, the blue of the third".[3]
Ravindra Bapat provided an alternative proof of this generalization, based on the permutation variant of Sperner's lemma.[4]
Note: the original KKM lemma follows from the permutation lemma by simply picking n identical coverings.
Connecting-sets
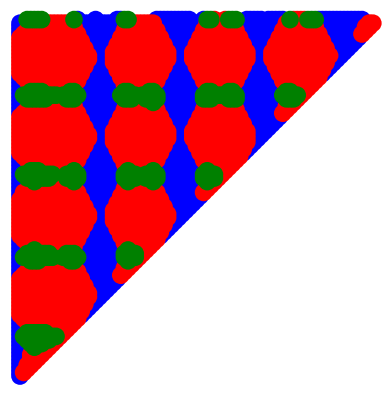
A connecting set of a simplex is a connected set that touches all n faces of the simplex.
 in which no
in which no contains a connecting set.
contains a connecting set. even touches all n faces. However, there are generalized-KKM-coverings that are not KKM-coverings. An example is illustrated at the right. There, the red set touches all three faces, but it does not contain any connecting-set, since no connected component of it touches all three faces.
even touches all n faces. However, there are generalized-KKM-coverings that are not KKM-coverings. An example is illustrated at the right. There, the red set touches all three faces, but it does not contain any connecting-set, since no connected component of it touches all three faces. ).
).The connecting-set variant also has a permutation variant, so that both these generalizations can be used simultaneously.
The KKMS theorem
The KKMS theorem is a generalization of the KKM lemma by Lloyd Shapley. It is useful in economics, especially in cooperative game theory.[6]
 sets - indexed by the nonempty subsets of
sets - indexed by the nonempty subsets of . For any
. For any , theconvex hullof the vertices corresponding to
, theconvex hullof the vertices corresponding to should becoveredby
should becoveredby .
. , such that the intersection of sets indexed by
, such that the intersection of sets indexed by is nonempty:[7]
is nonempty:[7]
 of subsets of
of subsets of is called balanced if, for each subset
is called balanced if, for each subset , we can select a weight
, we can select a weight , such that, for each element
, such that, for each element , the sum of weights of all subsets that contain
, the sum of weights of all subsets that contain is exactly 1. For example, suppose
is exactly 1. For example, suppose . Then:
. Then:The collection {{1}, {2}, {3}} is balanced: choose all weights to be 1. The same is true for any collection in which each element appears exactly once, such as the collection {{1,2},{3}} or the collection { {1,2,3} }.
The collection {{1,2}, {2,3}, {3,1}} is balanced: choose all weights to be 1/2. The same is true for any collection in which each element appears exactly twice.
The collection {{1,2}, {2,3}} is not balanced, since for any choice of positive weights, the sum for element 2 will be larger than the sum for element 1 or 3, so it is not possible that all sums equal 1.
The collection {{1,2}, {2,3}, {1}} is balanced: choose .
 , for
, for . Construct a KKMS covering
. Construct a KKMS covering as follows:
as follows:whenever ( is a singleton that contains only element ).
otherwise.
 implies the KKMS condition on the new covering
implies the KKMS condition on the new covering . Therefore, there exists a balanced collection such that the corresponding sets in the new covering have nonempty intersection. But the only possible balanced collection is the collection of all singletons; hence, the original covering has nonempty intersection.
. Therefore, there exists a balanced collection such that the corresponding sets in the new covering have nonempty intersection. But the only possible balanced collection is the collection of all singletons; hence, the original covering has nonempty intersection.Reny and Wooders proved that the balanced set can also be chosen to be partnered.[11]